What is Pi?

Did somebody say pie?! Well not that kind of pie. Get ready to dig into the Mathematics of STEM with this hands-on activity to prove the constant Pi.

Print this Experiment

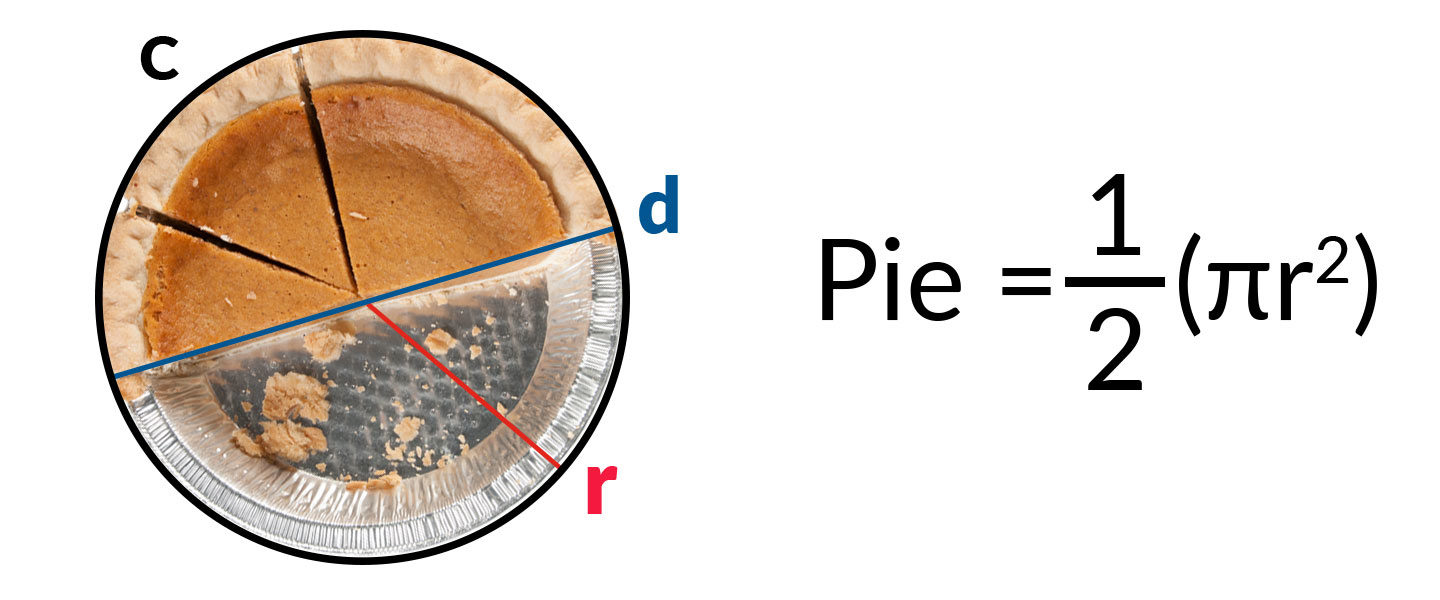
Pi (π) is the constant used in equations to find all of the dimensions of circles and spheres, but where did it come from? With a few easy activities you can discover the geometric constant that we celebrate each March 14th, and learn how it’s used in math AND science!

The other trait that Pi is known for is that it is an irrational number. This means that the number of digits following the decimal point is infinite, and will never repeat. Here’s the first few, so you can start memorizing!

3.141592653589793238462643383279502884197169399375105820974944592307816406286

Here's What You'll Need

  • Printed circle template
  • Thick piece of paper or cardboard
  • String or ribbon (~ 2ft)
  • Ruler
  • Scissors
  • Calculator (optional)

Let's Try It

  1. Download this Circle Template and print it on a thick piece of paper. Then cut out along the outline of your circle.

    If you don’t have thick paper available, use a glue stick to attach your template to card board such as from a cereal box. Then cut around the outline of the circle through both layers.

  2. Wrap the string around the cutout, following closely along the border, and trim it to the exact length of the circumference.

  3. Measure the length of the string you just cut. It should be approximately 22 inches (55.88 cm).

  4. Now measure the diameter of the circle template. You can find the diameter by measuring from one side of the circle, going through the center, all the way to the other side of the circle.

  5. This is the cool part where the magic happens. You can use measuring, division, or algebra to prove Pi.

    • Measuring: Measure how many times your diameter goes into your circumference. It should go in 3 times, and then leave 1 inch left on your 22 inch string. Or 3.14 times (approximately)
    • Divide: Divide the length of your long string, by the length of the diameter: 22 ÷ 7 = 3.14
    • Algebra: Circumference is diameter times Pi (C = dπ): 22 = 7x , x=3.14

How Does It Work

Looking for a more technical explanation to use? This is how you might see it in a calculus book, and how it was originally proven by Archimedes, before he knew a way to calculate the circumference of a circle.

  1. Print a fresh copy of the circle template.
  2. Draw a perfect square around the outside of the circle, touching in exactly 4 places (these lines are called tangents).
  3. Then connect one corner to the opposite by passing through the center of the circle.
  4. Now, draw a perfect square inside the circle, touching in exactly 4 places (these lines are called chords).
  5. Connect one corner to the opposite by passing through the center of the circle again. Your picture should look much like this image
  6. Now, we need to normalize our drawing by stating that the diameter is exactly 1 unit. So think of the perimeter of the large box as 4 units. We know that the diameter is 1 unit, so to find the length of a side for the small square use Pythagorean theorem: a2 + b2 =c2
    Since a and b are the same length, and we know the hypotenuse is 1, we can calculate:
    2a2 = 1 or a = √(1/2)
    Therefore the length of the sides is .7 units, and the perimeter would be 2.8.
  7. To find the circumference of the circle, we would then take the average between these two perimeters: (2.8 + 4) ÷ 2 = 3.4

Of course, 3.4 is not the 3.14 that Pi is calculated to be today, but to get closer and closer to the most accurate circumference, mathematicians use hexagons, octagons, or even decagons. The more sides that the internal and external shapes have, the more accurate the measurement gets, and the closer you are to 3.14.

Take It Further

The unbelievable part of Pi that makes it the incredible number that it is, is that no matter what circle (or sphere) you do this with, you will always get 3.14. So, find something circular around the house or classroom. We tried it with a coffee mug, coaster, and CD to give you a few examples. Measure the circumference first, then find the diameter, and what do you get? Does it work if you use inches, centimeters, feet?

If you’re looking for a great way to do this on a white board, side walk, or a sheet of paper, try this variation:

  1. Roll your circle along a sheet of paper exactly one rotation, noting where it starts and stops. This will give you the circumference of the circle. (If you’re doing this outside, it works great to use a bicycle or car tire, just mark it chalk and roll it forward 1 rotation.)
  2. Then trace the outline of your circle along the length of your circumference. You should be able to draw 3 full circles with .14 of a dimeter remaining. (For a tire, just measure your diameter, and then line it up to the chalk along the ground.)

Word Wall

Area of a Circle (A) – The number of square units  inside of the circle. A = πr2

Chord – A line crossing from edge to edge in a circle, not necessarily through the center.

Circumference (C) – The distance around a curved geometric shape, in this instance a circle. C = 2πr

Diameter (d) – A line crossing from edge to edge through the center of a circle. d = 2r

Hypotenuse – The side of a triangle that is opposite the right angle.

Irrational Number – A real number that has infinite integers following the decimal point without repeating.

Normalize – The process of bringing a function back to its most basic (or normal) form.

Radius (r) – A line from the center of the circle to the edge. Exactly half of the diameter. r = d ÷ 2

Tangent – A line that touches a curve or circle at only one point.

Browse more experiments by concept:

Back to all experiments